Demo   Free videos       Math Tips, Tricks, & Anecdotes is a collection of 42 short videos of unusual facts, memory aids, humor, and general motivational techniques developed over an entire teaching career. Author Charles Cook reveals his secret techniques for helping students remember math facts and formulas all the way from things as mundane as the slope formula to why the integral sign is shaped as it is. Often told with a little dry humor, these tips and tricks can be applied all the way from Alg I through Calculus and often elicit the response... "I never thought of it that way! 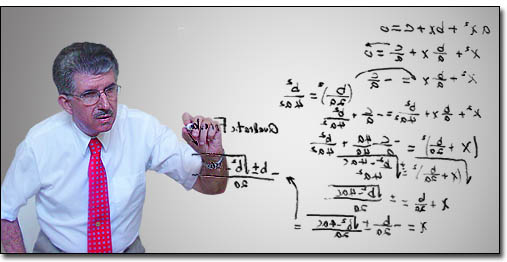"   Figured it out yet? What's Mr. Cook writing there? Reversed image        01 An easy way to remember how to change the base of a logarithm        02 A humorous look at the plural of focus. Makes it easy to remember        03 How an ellipse can eventually turn into a circle. What happens when the foci coalesce        04 Applications of the conic sections        05 A discussion of the difference between combinations and permutations.. selecting and ordering        06 Cross multiplication. Yes, it's a shortcut, but what is it, really?        07 Application of the distance formula. A simultaneous look at both the distance formula and the equation of a circle.        08 The relationship between a, b, and c for the ellipse and hyperbola. Normally, very confusing... an easy way to remember.        09 An absolutely stunning revelation. How to easily associate the following with x & y: horz, vert, domain, range, h, k, abscissa, ordinate, sin, cos, dependent, independent        10 Anything to the 0 power is 1. Oh yeah? Why is it true and what's the exception?        11 "Corn-bread are square". A sure-fire way to get them to remember the formula for the area of a circle        12 Origins of imaginary numbers... a historical perspective        13 More revelations about imaginary numbers. Students memorize that i2 is -1... but why?        14 Students often just go on auto-pilot and replace square root with the 1/2 power. Give them insight into why.        15 A special discussion of rationals and irrationals... with a little humor thrown in for good measure        16 Give students some hope that there is a practical use for the factoring of a2 - b2        17 Student confusion with linear inequalities.... tell them to "get out of the rain" and they'll never forget.        18 Is there a fourth dimension? What about people who live in just two-dimensions? A story from "Flat-Land".        19 What a big word!... orthogonal. What does it mean?        20 An interesting look at the arguments of sine and cosine in terms of frequency.        21 A neat way to think of sine and cosine... projection        22 The many ways (4) to define sine and cosine.. each useful in its own way        23 Can't remember if sin is x/r, y/r, etc. Let Oscar & Arthur help        24 Students are forever getting the two point form of the slope formula upside down. Never again with this little memory trick        25 A common technique for converting log & trig functions to their inverse forms        26 Scientific notation... moving the decimal place and adjusting the exponent..very confusing to students. an easy way to remember        27 Why are cos(a) = 1 and sin(a) = 0 approximately true for small angles? Makes them think about infinite series.        28 Explain why that button on your graphing calculator says x, t, & theta... yet when you push it, you only get x        29 The "art" of making 3-D drawings        30 Remember "wax on, wax off" from the Karate Kid movie? Apply it to matrices.        31 Why does replacing x with x - h and y with y - k translate the function? The ultimate, yet simple, explanation        32 Students think matrix multiplication is so abstract. Show them this simple, yet elegant practical application.        33 Where would a trillion seconds take us back in time?        34 A way to keep dependent and independent variables straight        35 Union and intersection: "I lost my keys..."        36 Determinants: multiply going down and it's positive, or is it negative? Easy way to remember.        37 PEMDAS: "Please excuse my dear Aunt Sally"        38 Contour lines on a map... normal and tangent lines        39 Why integral symbols have that particular shape        40 Coefficients of a binomial expansion. An easy way to get them.        41 An interesting comparison of rectangular and polar coordinates                       Demo            Free videos           Purchase